10th Maths Book Back Question & Answers – Chapter 1 Exercise 1.2:
Samacheer Kalvi 10th Standard Maths Book Back Questions with Answers PDF uploaded and the same given below. Class-tenth candidates and those preparing for TNPSC exams can check the Maths Book Back Answers PDF below. Samacheer Kalvi Class 10th Std Maths Book Back Answers Chapter 1 Exercise 1.2 Solutions are available below. Check the complete Samacheer Kalvi 10th Maths – Relations and Functions Ex 1.2 Book Back Answers below:
We also provide class 10th other chapters Maths Book Back One and Two Mark Solutions Guide on our site. Students looking for the 10th standard Maths – Relations and Functions Ex 1.2 Book Back Questions with Answer PDF
For the complete Samacheer Kalvi 10th Maths Book Back Solutions Guide PDF, check the link – Samacheer Kalvi 10th Maths Book Back Answers
Samacheer Kalvi 10th Maths Book Back Answers – Ex 1.2 Relations and Functions
Samacheer Kalvi 10th Maths Book Subject One Mark, Two Mark, Five Mark Guide questions and answers are below. Check Maths Book Back Questions with Answers. Take the printout and use it for exam purposes.
For Samacheer Kalvi 10th Maths Book PDF, check the link – 10th Maths Book PDF
Chapter 1
Exercise 1.2 Relations and Functions
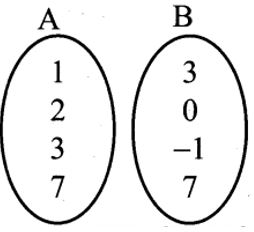
1. Let A = {1, 2, 3, 7} and B = {3, 0, -1, 7}, which of the following are related from A to B?
(i) R1 = {(2, 1), (7, 1)}
(ii) R2 = {(-1, 1)}
(iii) R3 = {(2, -1), (7, 7), (1, 3)}
(iv) R4 = {(7,-1), (0, 3), (3, 3), (0, 7)}
(i) A = {1, 2, 3, 7}, B = {3, 0,-1, 7}
Solu.:
R1 = {(2,1), (7,1)}
It is not a relation there is no element as 1 in B.
(ii) R2 = {(-1, 1)}
It is not [∵ -1 ∉ A, 1 ∉ B]
(iii) R3 = {(2, -1), (7, 7), (1, 3)}
It is a relation.
R4 = {(7,-1), (0, 3), (3, 3), (0, 7)}
It is also not a relation. [∵ 0 ∉ A]
2. Let A = {1, 2, 3, 4, ….., 45} and R be the relation defined as “is square of ” on A. Write R as a subset of A × A. Also, find the domain and range of R.
Solu.:
A = {1,2, 3, 4 . . . . 45}
The relation is defined as “is square of’
R = {(1,1) (2, 4) (3, 9)
(4, 16) (5,25) (6, 36)}
Domain of R = {1, 2, 3, 4, 5, 6}
Range of R = {1, 4, 9, 16, 25, 36}
3. A Relation R is given by the set {(x, y) /y = x + 3, x ∈ {0, 1, 2, 3, 4, 5}}. Determine its domain and range.
Solu.:
x = {0, 1, 2, 3, 4, 5}
y = x + 3
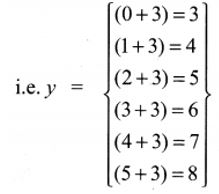
⇒ y = {3, 4, 5, 6, 7, 8}
R = {(x, y)}
= {(0, 3),(1, 4),(2, 5),(3, 6), (4, 7), (5, 8)}
Domain of R = {0, 1, 2, 3, 4, 5}
Range of R = {3, 4, 5, 6, 7, 8}
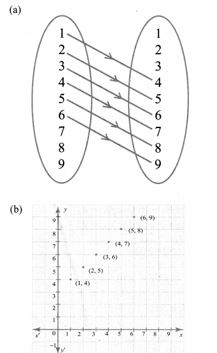
4. Represent each of the given relation by (a) an arrow diagram, (b) a graph and (c) a set in roster form, wherever possible.
(i) {(x, y)|x = 2y,x ∈ {2, 3, 4, 5},y ∈ {1, 2, 3, 4)
(ii) {(x, y)|y = x + 3, x, y are natural numbers <10}
Solu.:
(i){(x, y)|x = 2y, x ∈ {2, 3, 4, 5},y ∈ {1, 2, 3, 4}} R = (x = 2y)
2 = 2 × 1 = 2 4 = 2 × 2 = 4
(c) {(2, 1), (4, 2)}
(ii) {(x, y)|y = x + 3, x,+ are natural numbers <10}
x = {1, 2, 3, 4, 5, 6, 7, 8, 9} R = (y = x + 3)
y = {1, 2, 3, 4, 5, 6, 7, 8, 9}
R = {(1, 4), (2, 5), (3, 6), (4, 7), (5, 8), (6, 9)}
(c) R = {(1, 4), (2, 5), (3, 6), (4, 7), (5, 8), (6, 9)}
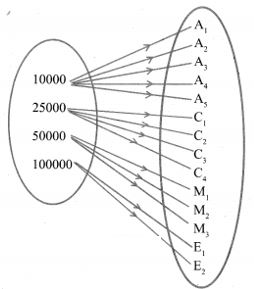
5. A company has four categories of employees given Assistants (A), Clerks (C), Managers (M), and an Executive Officer (E). The company provides Rs.10,000, Rs.25,000, Rs.50,000, and Rs.1,00,000 as salaries to the people who work in categories A, C, M, and E respectively. If A1, A2, A3, A4, and As were Assistants; C1, C2, C3, C4 were Clerks; M1, M2, M3 were managers, and E1, E2 were Executive officers and if the relation R is defined by xRy, where x is the salary given to person y, express the relation R through an ordered pair and an arrow diagram.
Solu.:
A – Assistants → A1, A2, A3, A4, A5
C – Clerks → C1, C2, C3, C4
D – Managers → M1, M2, M3
E – Executive officer → E1, E2
(a) R = {(10,000, A1), (10,000, A2), (10,000, A3),
(10,000, A4), (10,000, A5), (25,000, C1),
(25,000, C2), (25,000, C3), (25,000, C4),
(50,000, M1), (50,000, M2), (50,000, M3),
(1,00,000, E1), (1,00,000, E2)}
(b)
Other Important Links for 10th Maths Book Back Answers Solutions:
For 10th Maths Chapter 1 book back question and answers, check the link – Samacheer Kalvi 10th Maths Chapter 1 Relations and Functions
Click Here for the Complete Samacheer Kalvi 10th Book Back Solution Guide PDF – Samacheer Kalvi 10th Maths Book Back Answers