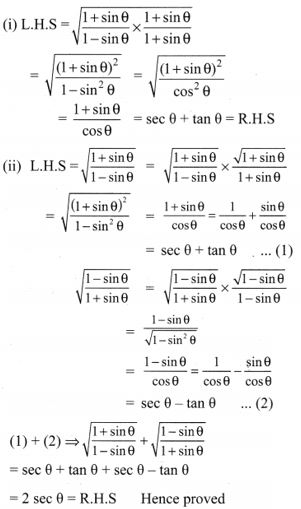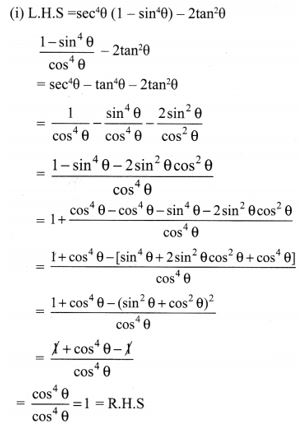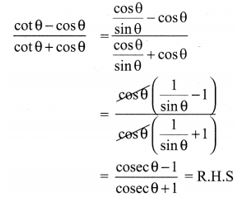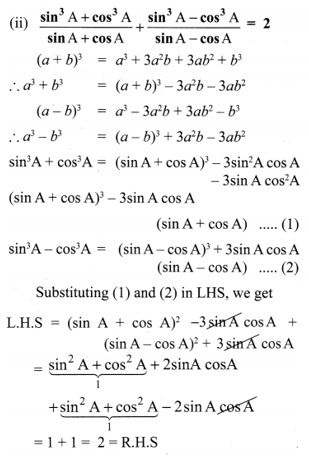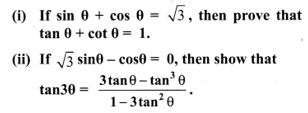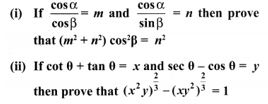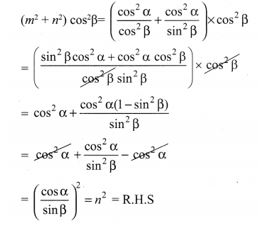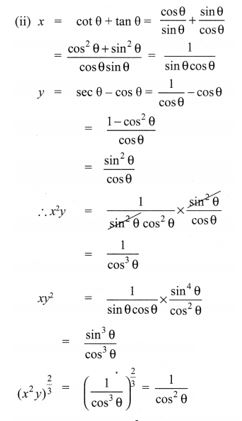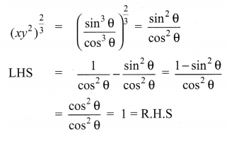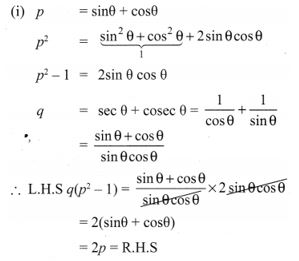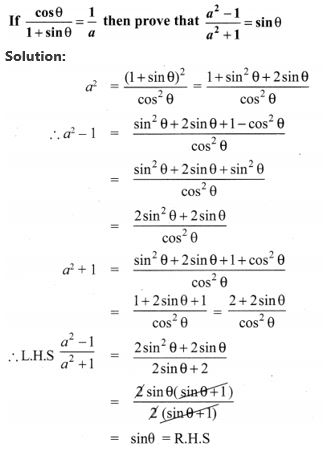10th Maths Book Back Question and Answers – Chapter 6 Exercise 6.1:

Samacheer Kalvi 10th Standard Maths Book Back Questions with Answers PDF uploaded and the same given below. Class-tenth candidates and those preparing for TNPSC exams can check the Maths Book Back Answers PDF below. Samacheer Kalvi Class 10th Std Maths Book Back Answers Chapter 6 Trigonometry Exercise 6.1 Solutions are available below. Check the complete Samacheer Kalvi 10th Maths – Trigonometry Ex 6.1 Book Back Answers below:

We also provide class 10th other units Maths Book Back One, Two, and Five Mark Solutions Guide on our site. Students looking for the 10th standard Maths Ex 6.1 – Trigonometry Book Back Questions with Answer PDF

For the complete Samacheer Kalvi 10th Maths Book Back Solutions Guide PDF, check the link – Samacheer Kalvi 10th Maths Book Back Answers

Samacheer Kalvi 10th Maths Book Back Answers – Ex 6.1 Trigonometry

Samacheer Kalvi 10th Maths Book Subject One Mark, Two Mark, Five Mark Guide questions and answers are below. Check Maths Book Back Questions with Answers. Take the printout and use it for exam purposes.

For Samacheer Kalvi 10th Maths Book PDF, check the link – 10th Maths Book PDF

Chapter 6

Exercise 6.1 Trigonometry

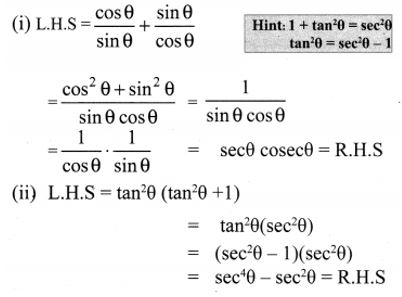
1. Prove the following identities.
(i) cot θ + tan θ = sec θ cosec θ
(ii) tan⁴θ + tan²θ = sec⁴θ – sec²θ
Solution:

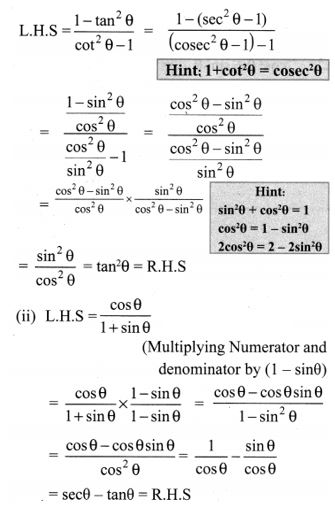
2. Prove the following identities

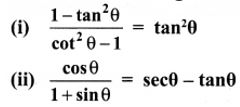
Solution:

3. Prove the following identities

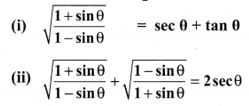
Solution:

4. Prove the following identities
(i) sec⁶θ = tan⁶θ + 3tan²θ sec²θ + 1
(ii) (sinθ + secθ)² + (cosθ + cosecθ)²= 1 + (secθ + cosecθ)²
Solu:

(i) L.H.S = sec⁶θ = (sec²θ)³ = (1 + tan²θ )³ = (tan²θ + 1)³
(a + b)³ = a³ + 3a²b + 3ab² + b³
= (tan²θ)³ + 3(tan²θ)² × 1 + 3 × tan²θ × 1² + 1
= tan⁶θ + 3tan²θ × (sec²θ – 1) + 3tan²θ + 1
= tan⁶θ + 3tan²θsec²θ – 3tan²θ + 3tan²θ +1
= tan⁶θ + 3tan²θ sec²θ + 1 = R.H.S

(ii) L.H.S = (sinθ + secθ )² + (cosθ + cosecθ)²
= sin²θ + 2sinθ secθ + sec²θ + cos²θ + 2cosθ cosecθ+ cosec²θ

5. Prove the following identities

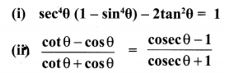
Solution:

(ii)

6. Prove the following identities

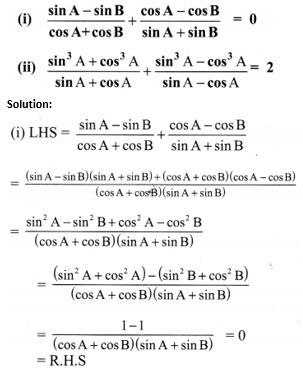
7.

Solution:

8.

Solution:
(i) LHS:

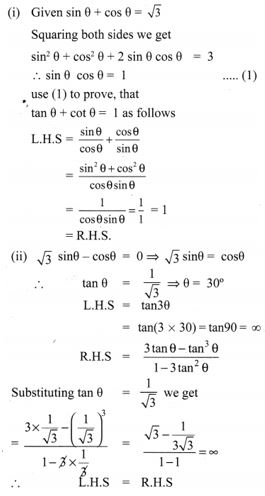
9. (i) If sinθ + cosθ = p and secθ + cosecθ = q then prove that q(p² – 1) = 2p
(ii) If sinθ(1 + sin²θ) = cos²θ, then prove that cos⁶θ – 4cos⁴θ + 8cos²θ = 4
Solution:

(ii) Given sinθ(1 + sin²θ) = cos²θ
Substitute sin²θ = 1 – cos²θ and take cos θ = c
squaring (1) on both sides we get
sin²θ(1 + sin²θ)² = cos⁴θ
(1 – c²)(1 + 1 – c²) = c⁴
(1 – c²)(2 – c²)² = c⁴
(1 – c²)(4 + c⁴– 4c²) = c⁴
4 + c⁴– 4c² – 4c² – c⁶ + 4c⁴ = c⁴
 -c^{6 +} 4c⁴ – 8c² = -4
c⁶ – 4c⁴ + 8c² = -4
ie cos 6θ – 4cos 4θ + 8cos²θ = 4 = RHS
∴ Hence proved

10.

Other Important Links for 10th Maths Book Back Answers Solutions:

For the 10th Maths Chapter 6 book back question and answers, check the link – Samacheer kalvi 10th Maths Chapter 6 Coordinate Geometry

Click here for the complete Samacheer Kalvi 10th Maths Book Back Solution Guide PDF – 10th Maths Book Back Answers