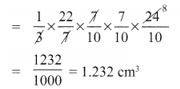10th Maths Book Back Question and Answers – Chapter 7 Exercise 7.3:
Samacheer Kalvi 10th Standard Maths Book Back Questions with Answers PDF uploaded and the same given below. Class-tenth candidates and those preparing for TNPSC exams can check the Maths Book Back Answers PDF below. Samacheer Kalvi Class 10th Std Maths Book Back Answers Chapter 7 Mensuration Exercise 7.3 Solutions are available below. Check the complete Samacheer Kalvi 10th Maths – Mensuration Ex 7.3 Book Back Answers below:
We also provide class 10th other units Maths Book Back One, Two, and Five Mark Solutions Guide on our site. Students looking for the 10th standard Maths Ex 7.3 – Mensuration Book Back Questions with Answer PDF.
For the complete Samacheer Kalvi 10th Maths Book Back Solutions Guide PDF, check the link – Samacheer Kalvi 10th Maths Book Back Answers
Samacheer Kalvi 10th Maths Book Back Answers – Ex 7.3 Mensuration
Samacheer Kalvi 10th Maths Book Subject One Mark, Two Mark, Five Mark Guide questions and answers are below. Check Maths Book Back Questions with Answers. Take the printout and use it for exam purposes.
For Samacheer Kalvi 10th Maths Book PDF, check the link – 10th Maths Book PDF
Chapter 7
Exercise 7.3 Mensuration
1. A vessel is in the form of a hemispherical bowl mounted by a hollow cylinder. The diameter is 14 cm and the height of the vessel is 13 cm. Find the capacity of the vessel.
Solution:
Diameter = 14 cm
Radius = 7 cm
Total height = 13 cm
Height of the cylindrical part = 13 – 7
= 6 cm
∴ Capacity of the vessel = Capacity of the cylinder + Capacity of the hemisphere.
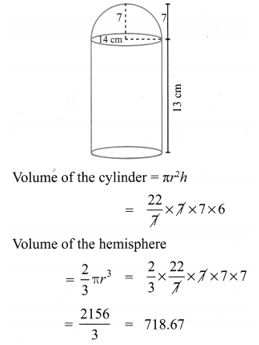
∴ The total volume = 924 + 718.67
The capacity of the vessel = 1642.67 cm3
2. Nathan, an engineering student was asked to make a model shaped like a cylinder with two cones attached at its two ends. The diameter of the model is 3 cm and its length is 12 cm. If each cone has a height of 2 cm, find the volume of the model that Nathan made.
Solution:
Volume of the model = Volume of the cylinder + Volume of 2 cones.

The volume of the model that Nathan made = 66 cm3
3. From a solid cylinder whose height is 2.4 cm and the diameter 1.4 cm, a cone of the same height and same diameter is carved out. Find the volume of the remaining solid to the nearest cm3.
Solution:
Volume of the cylinder = πr2h cu. units
Volume of the cone = 13πr2h cu. units
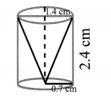
d = 1.4 cm, r = 0.7 cm = 710
h = 2.4 cm = 2410
Volume of the cylinder:
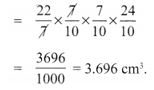
∴ Volume of the remaining solid = Volume of the cylinder – Volume of the cone
= 3.696 – 1.232
= 2.464
= 2.46 cm3
4. A solid consisting of a right circular cone of height 12 cm and radius 6 cm standing on a hemisphere of radius 6 cm is placed upright in a right circular cylinder full of water such that it touches the bottom. Find the volume of the water displaced out of the cylinder, if the radius of the cylinder is 6 cm and height is 18 cm.
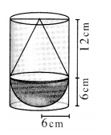
Solution:
Volume of water displaced out = Volume of the solid immersed in.
Volume of the solid = Volume of the cone + Volume of the hemisphere
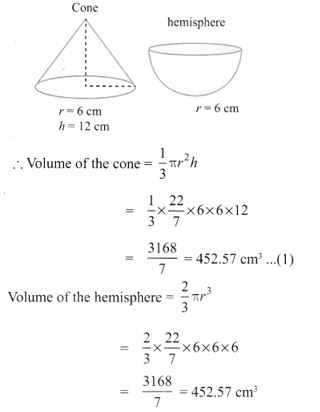
∴ The volume of water displaced out = Volume of the solid
= (1) + (2)
= 905.14 cm3
5. A capsule is in the shape of a cylinder with two hemispheres stuck to each of its ends. If the length of the entire capsule is 12 mm and the diameter of the capsule is 3 mm, how much medicine it can hold?
Solution:
Volume of medicine the capsule can hold = Volume of the cylinder + 2 volume of hemisphere
Volume of the cylinder part
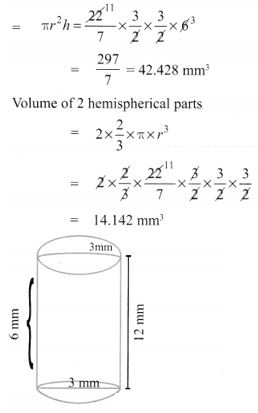
∴ The total volume = 56.571 mm3
∴ The volume of the medicine the capsule can hold = 56.57 mm3
6. As shown in the figure a cubical block of side 7 cm is surmounted by a hemisphere. Find the surface area of the solid.
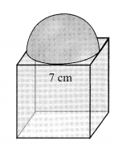
Solution:
Clearly, greatest diameter of the hemisphere is equal to the length of an edge of the cube is 7 cm.
Radius of the hemisphere = 72 cm
Now, total surface area of the solid = Surface area of the cube + Curved surface area of the hemisphere – Area of the base of the hemisphere.
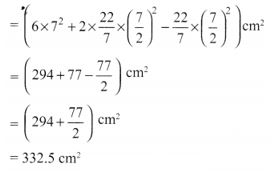
7. A right circular cylinder just enclose a sphere of radius r units.
Calculate
(i) the surface area of the sphere
(ii) the curved surface area of the cylinder
(iii) the ratio of the areas obtained in (i) and (ii).
Solution:
(i) Surface area of sphere = 4πr2 sq. units

8. A shuttle cock used for playing badminton has the shape of a frustum of a cone is mounted on a hemisphere. The diameters of the frustum are 5 cm and 2 cm. The height of the entire shuttle cock is 7 cm. Find its external surface area.
Solution:
The external surface area of the cock = Surface area of frustum + CSA of the hemisphere
Other Important Links for 10th Maths Book Back Answers Solutions:
For the 10th Maths Chapter 7 book back question and answers, check the link – Samacheer kalvi 10th Maths Chapter 7 Mensuration
Click here for the complete Samacheer Kalvi 10th Maths Book Back Solution Guide PDF – 10th Maths Book Back Answers