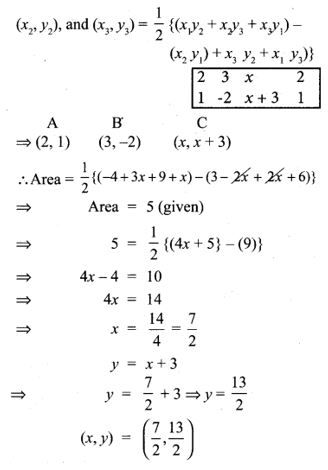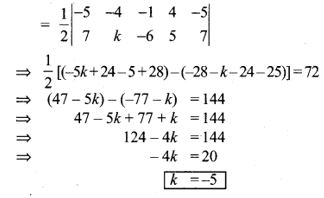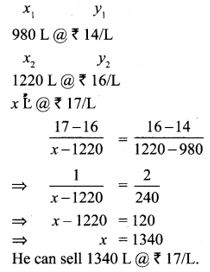10th Maths Book Back Question and Answers – Chapter 5 Exercise 5.5:
Samacheer Kalvi 10th Standard Maths Book Back Questions with Answers PDF uploaded and the same given below. Class-tenth candidates and those preparing for TNPSC exams can check the Maths Book Back Answers PDF below. Samacheer Kalvi Class 10th Std Maths Book Back Answers Chapter 5 Coordinate Geometry Exercise 5.5 Solutions are available below. Check the complete Samacheer Kalvi 10th Maths – Coordinate Geometry Ex 5.5 Book Back Answers below:
We also provide class 10th other units Maths Book Back One, Two, and Five Mark Solutions Guide on our site. Students looking for the 10th standard Maths Ex 5.5 – Coordinate Geometry Book Back Questions with Answer PDF
For the complete Samacheer Kalvi 10th Maths Book Back Solutions Guide PDF, check the link – Samacheer Kalvi 10th Maths Book Back Answers
Samacheer Kalvi 10th Maths Book Back Answers – Ex 5.5 Coordinate Geometry
Samacheer Kalvi 10th Maths Book Subject One Mark, Two Mark, Five Mark Guide questions and answers are below. Check Maths Book Back Questions with Answers. Take the printout and use it for exam purposes.
For Samacheer Kalvi 10th Maths Book PDF, check the link – 10th Maths Book PDF
Chapter 5
Exercise 5.5 Coordinate Geometry
Multiple choice questions:
1. The area of triangle formed by the points (-5, 0), (0, -5) and (5, 0) is
(1) 0 sq. units
(2) 25 sq.units
(3) 5 sq. units
(4) None of these
Solution:
(2) 25 sq. units
Hint:
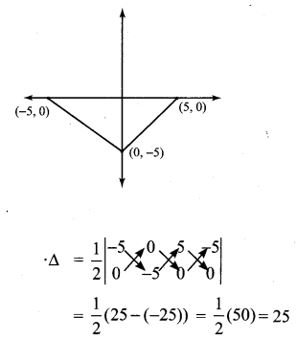
2. A man walks near a wall, such that the distance between him and the wall is 10 units.
Consider the wall to be the Y axis. The path traveled by the man is
(1) x = 10
(2) y = 10
(3) x = 0
(4) y = 0
Solution:
(1) x = 10
Hint:

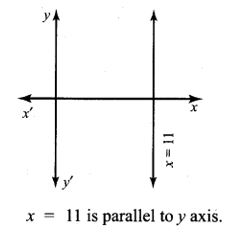
3. The straight line given by the equation x = 11 is
(1) parallel to X-axis
(2) parallel to Y-axis
(3) passing through the origin
(4) passing through the point (0,11)
Solution:
(2) Parallel to the y-axis
Hint:
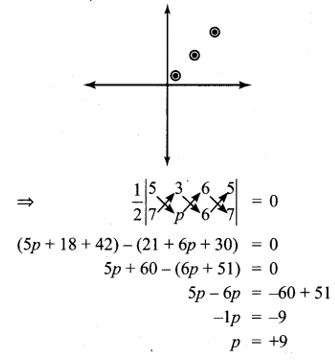
4. If (5, 7), (3, p), and (6, 6) are collinear, then the value of p is
(1) 3
(2) 6
(3) 9
(4) 12
Solution:
(3) 9
If (5, 7), (3, p) and (6, 6) are collinear ∆ = 0
5. The point of intersection of 3x – y = 4 and x + y = 8 is
(1) (5, 3)
(2) (2, 4)
(3) (3, 5)
(4) (4, 4)
Solution:
(3) (3, 5)]
Hint:
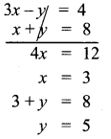
∴ The point of intersection is (3, 5)
6. The slope of the line joining (12, 3), (4, a) is 18. The value of ‘a’ is
(1) 1
(2) 4
(3) -5
(4) 2
Solution:
(4) 2
Hint:

7. The slope of the line which is perpendicular to a line joining the points (0, 0) and (-8, 8) is
(1) -1
(2) 1
(3) 13
(4) -8
Solution:
(2) 1
Hint:
The slope of the line joining the points (0, 0) and (-8, 8) is
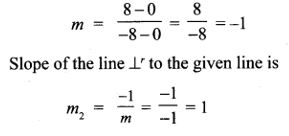
8. If slope of the line PQ is 13√ then the slope of the perpendicular bisector of PQ is
(1) 3–√
(2) −3–√(3)
(3) 13√
(4) 0
Solution:
(2) −3–√(3)
9. If A is a point on the Y-axis whose ordinate is 8 and B is a point on the X-axis whose abscissa is 5 then the equation of the line AB is
(1) 8x + 5y = 40
(2) 8x – 5y = 40
(3) x = 8
(4) y = 5
Solution:
(1) 8x + 5y = 40
Hint:

10. The equation of a line passing through the origin and perpendicular to the line 7x – 3y + 4 = 0 is
(1) 7x – 3y + 4 = 0
(2) 3x – 7y + 4 = 0
(3) 3x + 7y = 0
(4) 7x – 3y = 0
Solution:
(3) 3x + 7y = 0
11. Consider four straight lines
(i) l1 : 3y = 4x + 5
(ii) l2: 4y = 3x – 1
(iii) l3: 4y = 3x = 7
(iv) l4 : 4x + 3y = 2
Which of the following statement is true?
(1) l1 and l2 are perpendicular
(2) l1 and l4 are parallel
(3) l2 and l4 are perpendiculars
(4) l2 and l3 are parallel
Solution:
(3) l2 and l4 are perpendiculars
12. A straight line has equation 8y = 4x + 21. Which of the following is true
(1) The slope is 0.5 and the y-intercept is 2.6
(2) The slope is 5 and the intercept is 1.6
(3) The slope is 0.5 and the y-intercept is 1.6
(4) The slope is 5 and the intercept is 2.6
Solution:
(1) The slope is 0.5 and the y-intercept is 2.6
13. When proving that a quadrilateral is a trapezium, it is necessary to show ………………
(1) Two sides are parallel.
(2) Two parallel and two non-parallel sides.
(3) Opposite sides are parallel.
(4) All sides are of equal length.
Answer:
(2) Two parallel and two non-parallel sides.
14. When proving that a quadrilateral is a parallelogram by using slopes you must find
(1) The slopes of two sides
(2) The slopes of two pairs of opposite sides
(3) The lengths of all sides
(4) Both the lengths and slopes of two sides
Solution:
(2) The slopes of two pair of opposite sides
15. (2, 1) is the point of intersection of two lines.
(1) x – y – 3 = 0; 3x – y – 7 = 0
(2) x + y = 3; 3x + y = 7
(3) 3x + y = 3; x + y = 7
(4) x + 3y – 3 = 0; x – y – 7 = 0
Answer:
(2) x + y = 3; 3x + y = 7
Hint:
Substitute the value of x = 2 and y = 1 in the given equation.
(1) ⇒ x – y – 3 = 0
2 – 1 – 3 = 0
2 – 4 = 0
– 2 ≠ 0
not true
3x – y – 7 = 0
3(2) – 1 – 7 = 0
6 – 8 = 0
-2 ≠ 0
not true
(2) ⇒ x + y = 3
2 + 1 = 3
3 = 3
True
3x + y = 7
3(2) + 1 = 7
6 + 1 = 7
7 = 7
True
∴ (2, 1) is the point of intersection
(3) ⇒ 3x + y = 3
3(2) + 1 = 3
6 + 1 = 3
7 = 3
not true
x + y = 7
2 + 1 = 7
3 = 7
not true
(4) ⇒ x + 3y – 3 = 0
2 + 3 – 3 = 0
5 – 3 = 0
2 ≠ 0
not true
x – y – 7 = 0
2 – 1 – 7 = 0
2 – 8 = 0
-6 ≠ 0
not true
Unit 5
Exercise 5
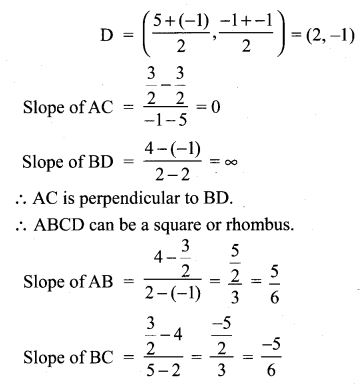
- PQRS is a rectangle formed by joining the points P(-1, -1), Q(-1, 4), R(5, 4) and S(5, -1). A, B, C, and D are the mid-points of PQ, QR, RS, and SP respectively. Is the quadrilateral ABCD a square, a rectangle, or a rhombus? Justify your answer.
Solution:
A, B, C, and D are midpoints of PQ, QR, RS & SP respectively.
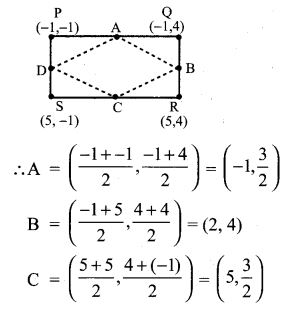
∴ AB and BC are not perpendicular
⇒ ABCD is rhombus as diagonals are perpendicular and sides are not perpendicular.
2. The area of a triangle is 5 sq. units. Two of its vertices are (2, 1) and (3, -2). The third Vertex is (x, y) where y = x + 3 . Find the coordinates of the third vertex.
Solution:
Area of triangle formed by points (x1, y1),
3. Find the area of a triangle formed by the lines 3x + y – 2 = 0, 5x + 2y – 3 = 0 and 2x – y – 3 = 0
Solution:
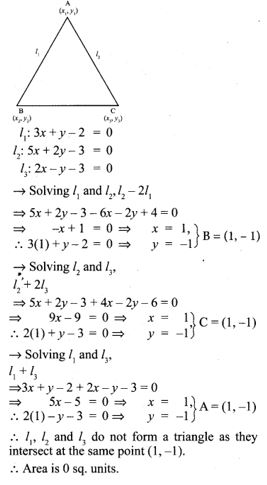
4. If vertices of a quadrilateral are at A(-5, 7), B(-4, k) , C(-1, -6) and D(4, 5) and its area is
72 sq.units. Find the value of k.
Solution:
5. Without using the distance formula, show that the points (-2, -1), (4, 0), (3, 3) and (-3, 2) are vertices of a parallelogram.
Solution:
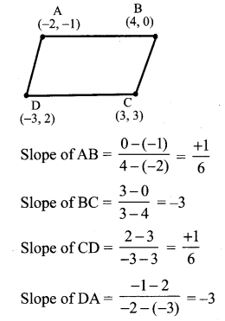
The slope of AB = Slope of CD
The slope of BC = Slope of DA
Hence ABCD forms a parallelogram.
6. Find the equations of the lines, whose sum and product of intercepts are 1 and -6 respectively.
Let the intercepts be x1, y1 respectively
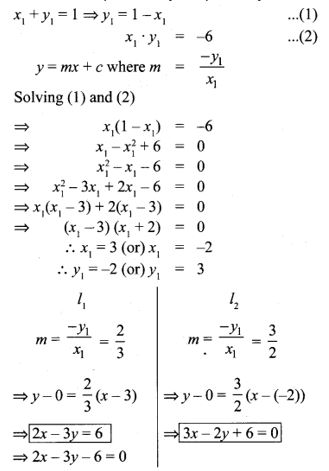
7. The owner of a milk store finds that, he can sell 980 litres of milk each week at ₹ 14/litre and 1220 litres of milk each week at ₹ 16 litre. Assuming a linear relationship
between selling price and demand, how many litres could he sell weekly at ₹ 17/litre?
Solution:
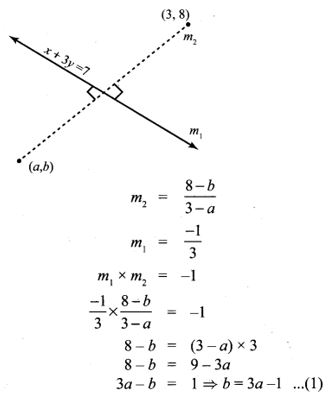
8. Find the image of the point (3, 8) with respect to the line x + 3y = 7 assuming the line to be a plane mirror.
Solution:
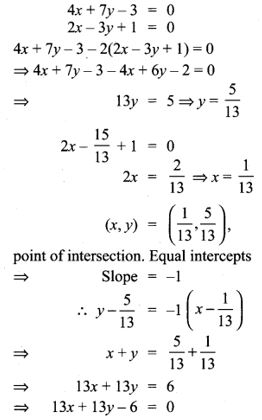
9. Find the equation of a line passing through the point of intersection of the lines 4x + 7y – 3 = 0 and 2x – 3y + 1 = 0 that has equal intercepts on the axes.
Solution:
4x + 7y – 3 = 0
2x – 3y + 1 = 0
4x + 7y – 3 – 2(2x – 3y + 1) = 0
10. A person standing at a junction (crossing) of two straight paths represented by the equations 2x – 3y + 4 = 0 and 3x + 4y – 5 = 0 seek to reach the path whose equation is 6x – 7y + 8 = 0 in the least time. Find the equation of the path that he should follow.
Solution:

Other Important Links for 10th Maths Book Back Answers Solutions:
For 10th Maths Chapter 5 book back question and answers, check the link – Samacheer kalvi 10th Maths Chapter 5 Coordinate Geometry
Click here for the complete Samacheer Kalvi 10th Maths Book Back Solution Guide PDF – 10th Maths Book Back Answers